
Integrating curvatures over beam length, the deflection, at some point along x-axis, should also be reversely proportional to I. Moment of Inertia Moment of inertia, also called the second moment of area, is the product of area and the square of its moment arm about a reference axis. Therefore, it can be seen from the former equation, that when a certain bending moment M is applied to a beam cross-section, the developed curvature is reversely proportional to the moment of inertia I. Moment of inertia can be defined by the equation The moment of inertia is the sum of the masses of the particles making up the object multiplied by their respective distances squared from the axis of rotation. V b A a y I 'y' Shear Force z x y V y 'x' Shear Force z x y V x V A y I b b a g Note : The maximum shear stress for common cross sections are: Cross Section : Cross Section : Rectangular: max 3 2 V. The polar moment of inertia may be found by taking the sum of the moments of inertia about two perpendicular axes lying in the plane of the cross-section and passing through this point. The moment of inertia of an object rotating about a particular axis is somewhat analogous to the ordinary mass of the object. Where Ixy is the product of inertia, relative to centroidal axes x,y (=0 for the rectangular tube, due to symmetry), and Ixy' is the product of inertia, relative to axes that are parallel to centroidal x,y ones, having offsets from them d_. distance between the two centroids Area moment of inertia of entire cross section about an axis pependicular to V. Where I' is the moment of inertia in respect to an arbitrary axis, I the moment of inertia in respect to a centroidal axis, parallel to the first one, d the distance between the two parallel axes and A the area of the shape, equal to bh-(b-2t)(h-2t), in the case of a rectangular tube.įor the product of inertia Ixy, the parallel axes theorem takes a similar form:
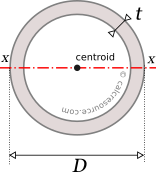
The so-called Parallel Axes Theorem is given by the following equation:
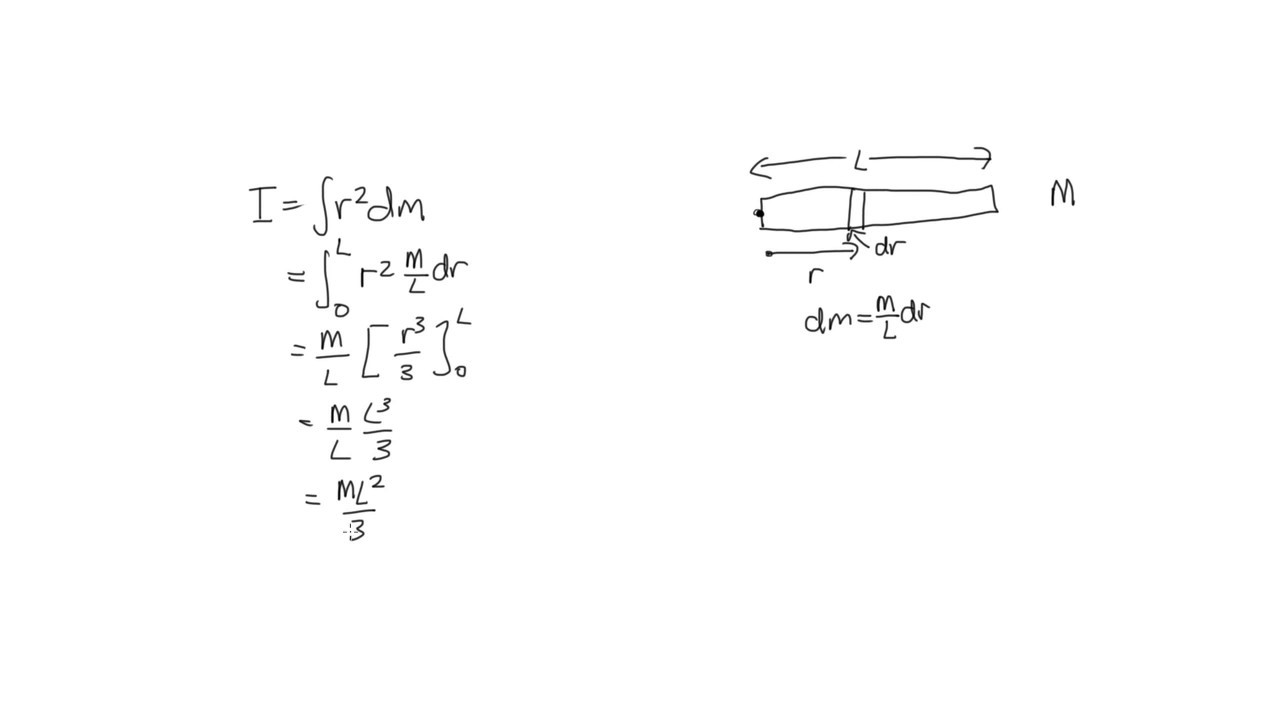
the mass moment of inertia of the tube and the rod and found that the tube and rod's mass moment of inertia are the same, ( 12-lbm-in2). The moment of inertia of any shape, in respect to an arbitrary, non centroidal axis, can be found if its moment of inertia in respect to a centroidal axis, parallel to the first one, is known. I am looking, which is stiffer the tube or the rod and lets say that the lengths are 12' long for both tube and rod and both weigh the same 1-lbm.


 0 kommentar(er)
0 kommentar(er)
